Table of Contents
ToggleJoule Thomson effect is an experimentally verified statement given by Joule and Thomson and later by Lord Kelvin, they observed the cooling effect when compressed gas is forced to pass through a porous plug into a low-pressure region. The Joule-Thomson Effect or Joule-Kelvin Effect is the phenomenon of dropping temperature when gas is forced to expand adiabatically from a high-pressure zone to a low-pressure region.
Joule Thomson effect
It states that “ when real gases are allowed to pass adiabatically through a porous plug from a region of higher pressure to a region of lower pressure, there is a sudden drop in their temperature except for hydrogen and helium.
The Joule-Thomson effect is illustrated schematically in the following figure.
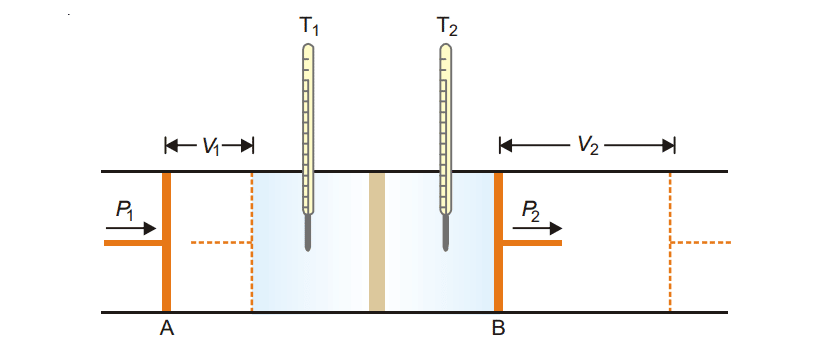
It consists of two frictionless pistons A and B, fitted on either side of the pistons plug. A real gas is kept between piston A and B at different pressure P1 and P2 such that P1 > P2. The real gas is allowed to expand from a high-pressure region into a lower pressure region through a porous plug. The temperature of both compartments is measured. A fall in temperature has been observed in gases except for hydrogen and helium.
Show that joule Thomson effect is an isenthalpic process
Work done by the piston A on the gas= -P1V1 ( W is negative )
Similarly, work done on the piston B = + P2V2 ( W is positive).
Now, total work done W= (-P1V1) + P2V2= P2V2 – P1V1 ……………………………………..(i)
Now, according to the first law of thermodynamics,
∆E = q- W
Since, expansion is adiabatic, q=0
∆E = – W ………………………………………………..(ii)
From equations (i) and (ii),
∆E= – (P2V2 – P1V1)
E2 – E1= P1V1 – P2V2
E2 + P2V2 = E1+ P1V1
Since enthalpy(H) = E + PV
So, H2=H1
H2– H1 = 0
∆H= 0 ……………………………………………..(iii)
Thus, the Joule-Thomson process is an isoenthalpic process.
Joule Thomson coefficient
Joule- Thomson coefficient is defined as the temperature change in degrees produced per atmospheric drop in pressure on passing a gas through the porous plug under the condition of constant enthalpy. It is denoted by μ. Mathematically, it is given by
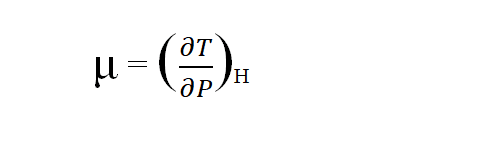
There are three conditions of the joule Thomson coefficient.
- If the value of μ = +ve then the cooling effect is observed.
- If μ= 0, then gas shows neither cooling nor heating effect.
- If μ= -ve then gas shows heating effect.
Inversion of temperature
The temperature at which a gas shows neither cooling nor heating effect on adiabatic expansion is called inversion of the temperature of the gas. At inversion temperature, the value of μ is zero
The inversion temperature for the hydrogen is -80ºC and above the inversion temperature, μ is negative. Thus, at room temperature hydrogen warms on expansion instead of cooling. A similar case for helium can be observed. Therefore, instead of cooling, the heating effect is seen when the joule Thomson experiment is carried out for these gases.
Joule-Thomson effect youtube video
References
- P. Atkins and J. de Paula, Atkins’ Physical Chemistry, (10th Edition), Indian edition,
Oxford University Press, 2014 - Arun Bahl, B. S. Bahl & G. D. Tuli, Essentials of Physical Chemistry, S. Chand and Company Ltd., New Delhi, 2012